
What is Proportional Integral Derivative?
Process Control emerged as a vital technology as far back as the 1940s when a standard tool for controlling processes like Generator Governors became a necessity. While many loop controls have made their way to the automation industry throughout the years, one has kept its ground: Proportion Integral Derivative Controller (PID). Today, 95% of all control loops are of PID type, being used in all areas as far as control is concerned.
PID controllers have thrived over the years in the face of several changes to technology from mechanics to pneumatics to microprocessors. The latest technology in use (transistor based microprocessors) have had a dramatic influence over PID controllers. Almost all PID controllers in use today are microprocessor based, giving them huge processing skills, extreme customizability and robustness.
A control loop is one that has a feedback mechanism in place to correct any error between measured process variable and a set value. A special-purpose computer called controller is put in place to apply the rectifying efforts through an actuator to drive the process variable up or down. An instant example would be a home furnace that drives the heat up or down depending on the readings given by thermostat.
At the industrial end, a PID controller:
The process is repeated until the error remains in place.
Based on the ideal or International Society of Automation standards, a PID controller has a PID algorithm that works on the following principle:
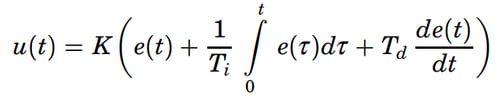
Where P is the controller gain, Ti is the integral time & Td is the derivative time.
Gain is defined as the percentage by which the error signal will increase or decrease as it passes through the controller. The gain value holds great importance and setting it correctly is vital for reduced errors in the output. Generally, a PID controller with a high gain is bound to generate aggressively correct output.
The integral time refers to an imagined sequence of events, where the error starts at zero and jumps to a certain value. A PID controller with a long integral time is more inclined towards proportional action than instantaneous action.
Finally, the derivate time shows the relative influence of the derivative term within the PID formula. Naturally, a ID controller with a long derivative time would be more inclined towards derivative action than proportional action.
When the first feedback controllers were introduced, they included just the proportional terms but when the integral term was introduced, operators observed that it made the controller intelligent at removing errors automatically. The derivative term was introduced after some time, and given the title of “rate control”.
The primary purpose of a PID controller is to prevent fluctuations in the output while eliminating error as soon as possible. Loop tuning is therefore an art, where the values of P, Ti and Td must be selected with careful considerations.
Tuning PID loops isn’t simple and requires techniques such as Ziegler-Nicholas Open and Closed loop ones. The rules of tuning change when:
Other techniques such as Cohen-Coon tuning rules have also made their place giving way to self-regulating processes governed by microprocessors. But in the end, Loop tuning becomes more of an art, requiring more experience from a Control Engineer than raw-knowledge.
Nonetheless, all modern process control systems wouldn’t be existent without PID controllers which are undoubtedly the base for all industrial automation technologies.
You may also be interested in reading:


